ProbabilityThe Monty Hall Problem

Welcome to the most spectacular game show on the planet! You now have a once-in-a-lifetime chance of winning a fantastic sports car which is hidden behind one of these three doors. Unfortunately, there are only goats behind the other two doors. Select one to make your choice!

Are you sure about that? You can still change your mind and select a different door…

I’m sure!

A great choice, but let me make life a little easier for you. I’ll open one of the other doors with a goat, so that there are only two doors left for you to pick from. Do you want to stick with your choice, or do you want to swap?

I want to stay!I want to swap!

Ok – let’s see how you did…

Open the doors…

Looks like you made the right choice. Congratulations, you just won a beautiful new sports car! Sorry – it seems like this time you only won a goat. But don’t worry, you can play again!

Replay game

If you play this game many times, you’ll notice that you’re more likely to win if you swapdon’t swap after the first door is opened, rather than sticking with your initial choice.

But how can this be – surely the car is equally likely to be behind each of the two remaining doors?

The explanation is very subtle. When you pick the initial door, the probability of being correct is 13 and the probability of being wrong is 23.

After the game master opens one of the other doors, the probability of being wrong is still 23, except now all this probability is on just one door. This means that swapping doors doublestripleshalves your chance of winning.

Even if this doesn’t seem very intuitive, we can prove that it is correct – simply by listing all different possibilities:

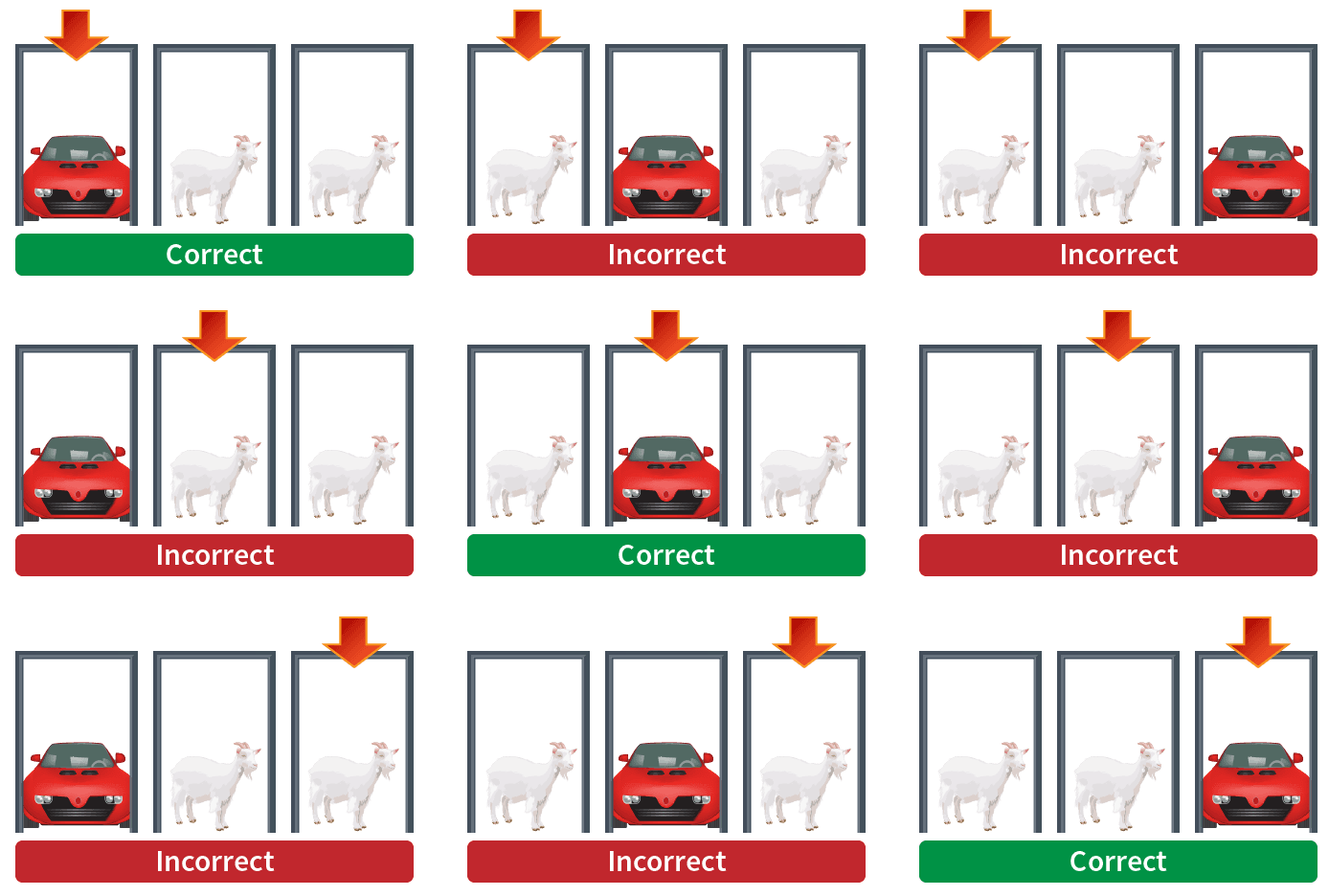
Out of the 9 possibilities, there are where you need you to switch doors, in order to win. This gives a chance of 69=23 like before.