Polygons and PolyhedraTessellations

Polygons appear everywhere in nature. They are especially useful if you want to tile a large area, because you can fit polygons together without any gaps or overlaps. Patterns like that are called tessellations.

Humans have copied many of these natural patterns in art, architecture and technology – from ancient Rome to the present. Here are a few examples:

Here you can create your own tessellations using regular polygons. Simply drag new shapes from the sidebar onto the canvas. Which shapes tessellate well? Are there any shapes that don’t tessellate at all? Try to create interesting patterns!

Examples of other students’ tessellations

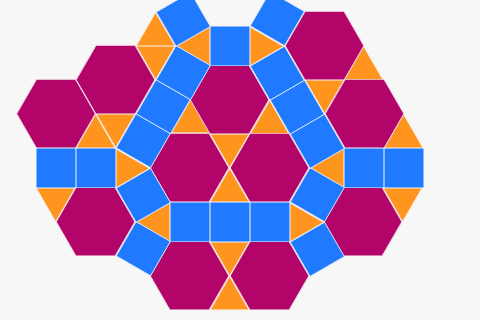
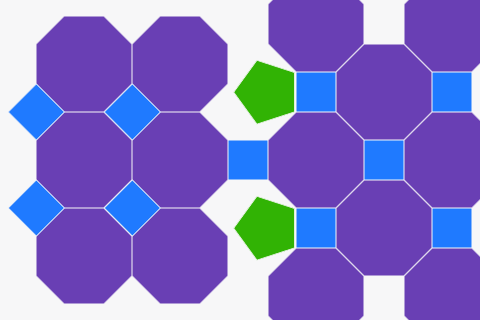
Tessellations from regular polygons

You might have noticed that some regular polygons (like squarespentagons) tessellate very easily, while others (like pentagonstriangleshexagons) don’t seem to tessellate at all.

This has to do with the size of their internal angles, which we learned to calculate before. At every vertex in the tessellation, the internal angles of multiple different polygons meet. We need all of these angles to add up to °, otherwise there will either be a gap or an overlap.

You can similarly check that, just like pentagons, any regular polygon with 7 or more sides doesn’t tessellate. This means that the only regular polygons that tessellate are triangles, squares and hexagons!

Of course you could combine different kinds of regular polygons in a tessellation, provided that their internal angles can add up to 360°:

Squares and triangles
90° + 90° + 60° + 60° + 60° = 360°

Squares and triangles
90° + 90° + 60° + 60° + 60° = 360°

Hexagons and triangles
120° + 120° + 60° + 60° = 360°

Hexagons and triangles
120° + 60° + 60° + 60° + 60° = 360°

Hexagons, squares and triangles
120° + 90° + 90° + 60° = 360°

Octagons and squares
135° + 135° + 90° = 360°

Dodecagons (12-gons) and triangles
150° + 150° + 60° = 360°

Dodecagons, hexagons and squares
150° + 120° + 90° = 360°

Tessellations from irregular polygons

We can also try making tessellations out of irregular polygons – as long as we are careful when rotating and arranging them.

Pentagons are a bit trickier. We already saw that regular pentagons don’t tessellatetessellate, but what about non-regular ones?

Here are three different examples of tessellations with pentagons. They are not regular, but they are perfectly valid 5-sided polygons:

Over time, mathematicians have only found 15 different kinds of tessellations with (convex) pentagons – the most recent of which was discovered in 2015.

Two years later, in 2017, Michaël Rao published a proof that there are no other possibilities, except the 15 that had already been found. Can you make a tessellation using all of them?

Tessellations in Art

Many artists, architects and designers use tessellations in their work. One of the most famous examples is the Dutch artist M. C. Escher. His works contain strange, mutating creatures, patterns and landscapes:

These artworks often look fun and effortless, but the underlying mathematical principles are the same as before: angles, rotations, translations and polygons. If the maths isn’t right, the tessellation is not going to work!

“Metamorphosis II” by M. C. Escher (1940)

Penrose Tilings

All the tessellations we saw so far have one thing in common: they are periodic. That means they consist of a regular pattern that is repeated again and again. They can continue forever in all directions and they will look the same everywhere.

In the 1970s, the British mathematician and physicist Roger Penrose discovered non-periodic tessellations – they still continue infinitely in all directions, but never look exactly the same. These are called Penrose tilings, and you only need a few different kinds of polygons to create one:

Penrose was exploring tessellations purely for fun, but it turns out that the internal structure of some real materials (like aluminium) follow a similar pattern. The pattern was even used on toilet paper, because the manufacturers noticed that a non-periodic pattern can be rolled up without any bulges.